On the Proof of the Existence of Nontotal Partial Degree and on the Turing Degree of Representative of This Partial Degree
Keywords:
e-reducibility, partial degree, partial computable function, Turing degreeAbstract
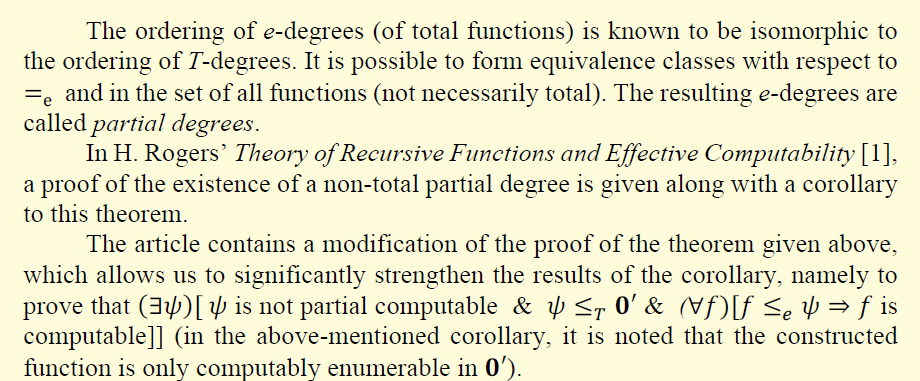
References
H. Rogers Jr., Theory of Recursive Functions and Effective Computability, McGraw-Hill, 1967.
R. M. Friedberg and H. Rogers Jr., Reducibility and completeness for sets of integers, Zeitschrift für mathematische Logik und Grundlagen der Mathematik 5, pp. 117–125, 1959.
J. Myhill, “Note on degrees of partial functions”, Proceedings of the American Mathematical Society 12, pp. 519–521, 1961.
A. L. Selman, “Arithmetical reducibilities”, I, Zeitschrift für mathematische Logik und Grundlagen der Mathematik 17, pp. 335–350, 1971.
M. I. Soskova, The theory of the enumeration degrees, definability, and automorphisms, Contemporary Logic and Computing. Adrian Rezus, editor. College publications, pp.706-730, 2020.
S. Lempp, Th. Slaman and M. I. Soskova, “Fragments of the theory of the enumeration degrees”, Advances of Mathematics, vol. 383, no. 4, pp.107686, 2021.
H. Ganchev, I. Kalimullin, J. S. Miller, and M. I. Soskova, “A structural dichotomy in the enumeration degrees”, J. Symb. Log., vol. 87, no. 2, pp. 527-544, 2022.
R.I. Soare, Recursively Enumerable Sets and Degree: A study of computable functions and computably generated sets, Perspectives in Mathematical Logic, Springer-Verlag, 1987.
Yu. T. Medvedev, Degrees of difficulty of the mass problem (in Russian), Doklady Academii Nauk SSSR, vol. 104, pp. 501-504, 1955.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2024 Arsen H. Mokatsian
This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
